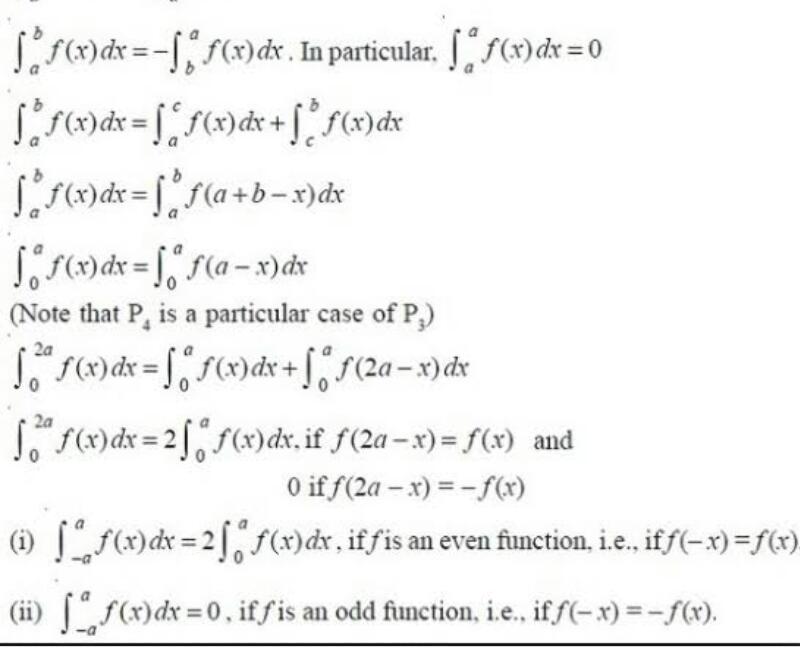
Week 7: Definite and indefinite integrals. Techniques of integration. (page 372 to 382, Exercise 5.2))

The definite integral is defined to be exactly the limit and summation that we looked at in the last section to find the net area between a function and the x -axis. Also note that the notation for the definite integral is very similar to the notation for an indefinite integral.

  1. The process of finding the indefinite integral is also called integration or integrating f(x). f ( x ) .
  2. The above definition says that if a function F is an antiderivative of f, then. ∫f(x)dx=F(x)+C. for some real constant C. C .
  3. Unlike the definite integral, the indefinite integral is a function.       Technique of integrations:           Integration by parts,   u-substitution,,  Reverse chain rule,   Partial fraction expansion,  Integration using trigonometric identities, Trigonometric substitution.

Download Files

stewart-calculas.pdf (32.22 MB )