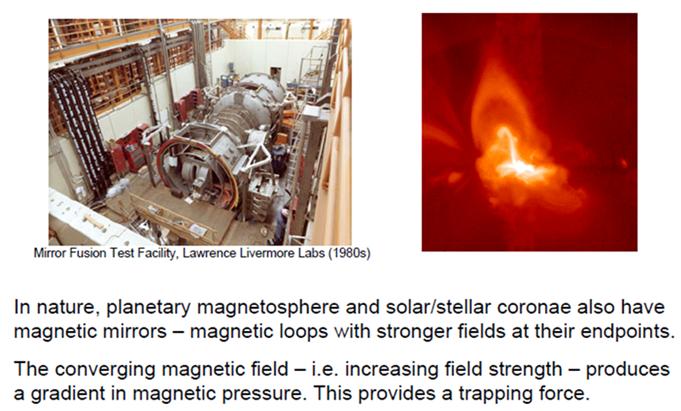
Week 7-8: Magnetic Mirrors, Non Uniform Electric field, Time varying electric field and Time varying B field.
When the fields are spatially nonuniform, the integration of the equation of motion can be a mathematical problem of great difficulty. In this case, since the equation of motion is nonlinear, the theory may become extremely involved, and rigorous analytic expressions for the charged particle trajectory cannot, in general, be obtained in closed form. Numerical methods of integration must be used in order to obtain all the details of the motion. There is one particularly important case, however, in which it becomes possible to obtain an approximate, but otherwise general, solution without recourse to numerical integration, if the details of the particle motion are not of interest. This is the case when the magnetic field is strong and slowly varying in both space and time, and when the electric field is weak. In a wide variety of situations of interest the fields are approximately constant and uniform, at least on the distance and time scales seen by the particle during one gyration about the magnetic field. This is the case for many laboratory plasmas, including those of relevance to the problem of controlled thermonuclear reactions, and also for a great number of astrophysical plasmas.
In first week we shall investigate the motion of a charged particle in a static magnetic field slightly inhomogeneous in space. The word slightly here means that the spatial variation of the magnetic field inside the particle orbit is small compared to the magnitude of B. In other words, we shall consider only magnetostatic fields whose spatial change in a distance of the order of the Larmor radius, is much smaller than the magnitude of the field itself.
In second week we shall analyze the motion of charged particles in the presence of time-varying fields. Initially, we consider a time-varying electric field and a constant magnetic field, both fields being spatially uniform. The assumption of a constant and spatially uniform B field is well justified if the externally applied magnetostatic field is much larger than the magnetic field associated with the time-varying E field. Also, the assumption of an electric field that is spatially uniform is valid if the charged particle cyclotron radius is much smaller than the scale length of the spatial variation of E.


